Remote Ready Biology Learning Activities has 50 remote-ready activities, which work for either your classroom or remote teaching.
Serendip is an independent site partnering with faculty at multiple colleges and universities around the world. Happy exploring!
Polygon | Circle | Ellipse | Stadium
This WEB page contains an interactive program that shows billiard motion in various shaped tables. Play with it and learn about chaos theory. Determine which tables have regular motion and which have chaotic motion.
The study of billiards provides an easy to understand introduction to the concepts of chaotic dynamical systems. In a dynamical system, an object moves according to a rule. A familiar example from physics is the planets which move according to the rules of gravity. One can consider the weather a dynamical system; there the weather moves, or changes, according to the rules of meteorology.
In mathematical billiards, we have one ball on a table that moves with constant speed forever. We pretend that there is no friction to slow the ball down. The rules of motion are as follows: a ball will move in a straight line until it hits the wall of the table. The ball will then bounce off the wall obeying the rule "angle of reflection equals angle of incidence". There are no pockets for the balls to bounce into.
As the ball continues to bounce off the walls, it may move in a regular, predictable fashion, or it may move in an irregular, chaotic fashion. Mathematicians try to understand how the shape of the table effects whether the billiard motion will be chaotic or regular.
Let's look at billiard motion inside a table bounded by straight
lines. We will start by looking at a square table. Click on
DRAW. You will see a ball move that has started at the center of the table
and moved in a diagonal direction. The ball moves for 10 bounces before the
program stops.
If you want to see the next 10 bounces, click on CONTINUE.
Does the motion look regular or chaotic to you? Do you notice any patterns
in the path the ball makes? ANSWER
You can start your own billiard path. Use the mouse to click at a point
inside the table. This is where the ball will start. Continue to hold the
mouse down and move the mouse in the direction you want the ball to move.
When you release the mouse button, you will see a line segment that is
in the direction the ball will move. Now click on DRAW to see the ball
move.
Try changing the number of bounces to see the ball bounce for a longer
period of time.
OTHER POLYGONS:
You can also change the number of sides of the polygon. Three sides will
give you billiards an equilateral triangle. Five sides will give a
pentagon, and so forth. Which ever number you choose, you will get a
regular polygon: all the sides will be the same length, all the angles will
be the same., and the vertices will lie on the unit circle.
Question: For different numbers of sides, figure out the interior angles
of the polygon. Figure out the length of the sides.
Try bouncing the ball in the square for 50 bounces. After a while, the
path begins to fill up the whole table and it becomes hard to keep track of
the balls progress. The ball is displaying a minor type of chaos: it is
going all over the table. But since this motion is made up of only a few
parallel lines, there is still a lot of regularity in the motion.
To help follow the motion and not get distracted by the clutter of the path
on the table, mathematicians invented an associated space, called the PHASE
SPACE, and study the motion of the billiard in the phase space.
Click on the button OPEN PHASE SPACE. You will see a blank
window open. This window is called the phase space. Start a ball moving on
the table. You will notice that each time the ball hits the wall of the
table, a dot is drawn in the phase space. Do a large number of bounces.
Do the dots in the phase space form a regular pattern or a chaotic pattern?
ANSWER
Since these dots form a regular pattern, we say that the billiard motion is
regular.
Can you find any PERIODIC ORBITS on the table?
Can you make a billiard trajectory on the square that will repeat itself
after 4 bounces (periodic orbit of period 4)?
You might want to figure out the correct starting point theoretically using
your algebra and geometry skills. Rather than starting the ball by using
the mouse to click, you can also enter the starting position of the ball
with numbers. In the data windows x and y , enter the starting position of
your ball. The direction of the ball can be set by giving a direction
vector v = (vx, vy). What this means is that for every vx units the ball
goes in the horizontal direction, it will travel vy units in the vertical
direction. Enter the values of vx and vy in the appropriate windows.
For different polygons, try finding periodic orbits of different periods.
Click on DRAW to see a ball moving inside a circular
billiard. Start the ball moving.
Do you notice a pattern being formed on the billiard table or is the motion
chaotic? ANS: Yes, as the ball bounces, an inner circle is created.
The ball traces out an inner circle. Each time the ball bounces, it just
grazes (is tangent to) this inner circle. We call such a shape a
CAUSTIC.
Can you find periodic orbits of period 2, 3, and 4 for this table?
To help follow the motion and not get distracted by the clutter of the path
on the table, mathematicians invented an associated space, called the phase space, and study the motion of the billiard in the phase space.
Look at the PHASE SPACE OF THE CIRCULAR BILLIARD. Do the dots form a
regular or chaotic pattern? ANSWER
In this phase space, the horizontal axis measures the point on boundary at
which the ball hits. This measure is taken to be the length along the
circle from the x axis (arc length). The vertical axis measures the
direction of the ball after it bounces off the wall. This direction is
measured by taking the angle between the direction of the ball and the
tangent to the circle.
Question: Can you find formulas for the new length and angle given the old
length and angle? ANSWER
You may determine the starting point and direction of the ball by clicking
in the phase space. The point on which you click corresponds to a point on
the table wall and a direction at the point. Watch the ball move.
Question: Is billiard motion in a circle regular or chaotic? Why?
ANSWER
Click on ELLIPSE to see billiards inside an ellipse.
Try different initial positions and directions for the ball. Do you see
patterns form? You should be able to see two different types of patterns
form.
TYPES OF PATTERNS: Some trajectories will produce an inner ellipse. We say
that these trajectories have a caustic that is an ellipse. Other
trajectories will outline an hyperbola. This is another type of caustic. Do
you see the two types of caustics? SAMPLE INITIAL CONDITIONS: the following
initial conditions will generate nice caustics.
Can you make periodic orbits with period 2 and 4?
Phase Space: The phase space picture for the ellipse is more complicated
than for the polygon or circle. A trajectory that generates an ellipse as
its caustic will cause a wavy curve of dots to be drawn in the phase space.
Trajectories that have hyperbolas as caustics will lead to a pair of
circles being drawn in the phase space. Each time the ball hits a wall, the
dot hops from one circle to another.
TYPES OF PATTERNS: Some trajectories will produce an inner ellipse. We say
that these trajectories have a caustic that is an ellipse. Other
trajectories will outline an hyperbola. This is another type of caustic. Do
you see the two types of caustics? SAMPLE INITIAL CONDITIONS: the following
initial conditions will generate nice caustics.
Can you make periodic orbits with period 2 and 4?
Phase Space: The phase space picture for the ellipse is more complicated
than for the polygon or circle. A trajectory that generates an ellipse as
its caustic will cause a wavy curve of dots to be drawn in the phase space.
Trajectories that have hyperbolas as caustics will lead to a pair of
circles being drawn in the phase space. Each time the ball hits a wall, the
dot hops from one circle to another.
Take a bunch of different initial dots in the phase space and generate
their paths (orbits). A nice pattern emerges. The phase space is filled up
by the two types of curves: the wavy curves at the top and bottom and the
circles in the middle. This picture is regular so we say the elliptical
billiard motion is regular. This billiard motion has the strongest type of
regularity: it is called INTEGRABLE.
The Stadium billiard consists of two half circles joined by straight lines.
Click here to play it.
QUESTIONS:
1. As you watch the ball moving around, does it follow a regular or
irregular pattern?
2. If you let the ball bounce for a large number of bounces, what happens?
3. In the phase space, what type of pattern do the dots make?
ANSWER
DISCUSSION: The idea of looking at billiard motion in a stadium shape was
introduced by the Soviet mathematician Bunimovich who is now a professor at
Georgia Tech University. Mathematicians have developed rigorous definitions
of chaotic motion, fancy terms such as ergodic, positive measure entropy,
Bernoulli. Bunimovich gave a mathematical proof that the billiard motion
was chaotic.
To Dynamical Systems |
To Billiards
POLYGONAL BILLIARDS:
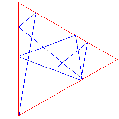
CIRCLE BILLIARDS:
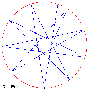
ELLIPTICAL BILLIARDS:

STADIUM BILLIARDS:
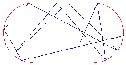
| Forum | Guest Exhibitions
| Serendip Home |
![]()
© by Serendip 1994-
- Last Modified:
Wednesday, 02-May-2018 10:53:24 CDT