Quantity, Quality, and Value:
A View From the Brain

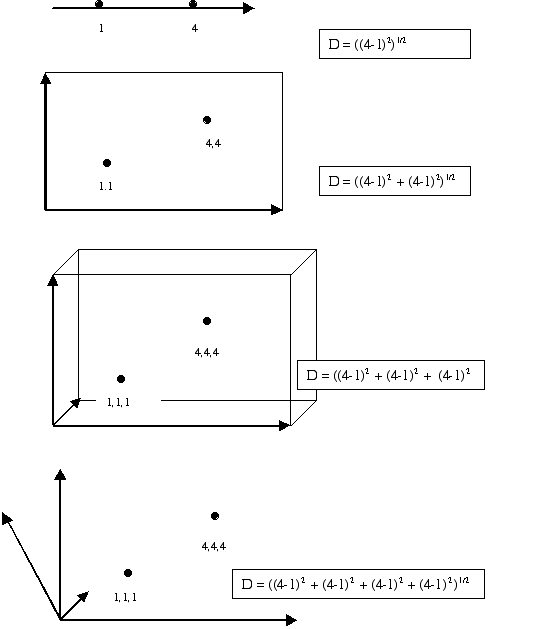
Figure 2
An Example of the Significance of Number and Mathematics

One can construct a plane from a line by drawing a second line perpendicular to the first, and a 3D volume by drawing a third line perpendicular to the first two. The distance between two points on a line, on a plane, and in a 3D volume can be calculated by formulae which follow a predictable pattern.

Though difficult or impossible to experience consciously, one can consciously continue the progression to four (or more) dimensions by adding additional lines perpendicular to those already present. The distance between points in spaces of higher dimensionality is perfectly coherently represented by extending the existing pattern for calculation of distance.